
讲座题目: A Win Ratio Approach to Comparing Ordinal or Non-Normal Outcomes in Clinical Research
讲座人: 王多劳教授,Liverpool School of Tropical Medicine
讲座摘要:
Clinical trials are often designed to compare continuous non-Normal outcomes. The conventional statistical method for such a comparison is a non-parametric Mann-Whitney test, which provides a P-value for testing the hypothesis that the distributions of both treatment groups are identical but does not provide a simple and straightforward estimate of treatment effect. For that, Hodges and Lehmann proposed estimating the shift parameter between two populations and its confidence interval. However, such a shift parameter does not have a straightforward interpretation and its confidence interval contains zero in some cases when Mann-Whitney test produces a significant result. To overcome the above problems, we introduce the use of the win ratio for analysing such data. Patients in the new and control treatment are formed into all possible pairs. For each pair the new treatment patient is labelled a “winner” or a “loser” if it is known who had the more favourable outcome. The win ratio is the total number of winners divided by the total numbers of losers. A 95% CI for the win ratio can be obtained using the bootstrap method. Statistical properties of the win ratio statistic are investigated using two real trial datasets and six simulation studies. Results show that the win ratio method has about the same power as the Mann-Whitney method. We recommend the use of the win ratio method for estimating the treatment effect (and CI) and the Mann-Whitney method for calculating the P-value for comparing continuous non-Normal outcomes when the amount of tied pairs is small.
讲座人简介:
王多劳教授,现任英国利物浦热带医学院生物统计教授,生物统计组主任、热带病临床研究中心副主任、Lancet评审、BMJ Open Heart统计副编辑,英国皇家统计学会会员。王多劳教授在临床试验设计、妇幼健康评价及数理统计方面具有深厚造诣,研究兴趣广泛,涉及统计理论方法、贝叶斯统计、妇幼健康、临床试验、流行性方法及转化医学等多个方面,先后主持多个大型研究项目的设计、分析工作,成果斐然,饱受赞誉,已先后在NEJM, Lancet,JAMA等著名期刊发表文章一百余篇, 出版专著十余部。
讲座题目:A Reaction-Diffusion Model of Vector-Borne Disease with Periodic Delays
讲座人: 赵晓强教授, Memorial University of Newfoundland
讲座摘要:
A vector-borne disease is caused by a range of pathogens, and transmitted to hosts through vectors. To investigate the multiple effects of the spatial heterogeneity, the temperature sensitivity of extrinsic incubation period (EIP) and intrinsic incubation period (IIP), and the seasonality on disease transmission, we propose a nonlocal reaction-diffusion model of vector-borne disease with periodic delays. We introduce the basic reproduction ratio R0 for this model and then establish a threshold type result on its global dynamics in terms of R0. In the case where all the coefficients are constants, we further obtain the global attractivity of the positive steady state when R0 is greater than one. We also carry out numerical simulations to study the malaria transmission in Maputo Province, Mozambique.
讲座人简介:
赵晓强教授1983年和1986年分别在西北大学数学系获学士和硕士学位,1990年1月在中国科学院应用数学研究所获博士学位,师从我国运动稳定性理论先驱秦元勋先生。1990年至1998在中科院应用数学研究所先后任助理研究员、副研究员、研究员。1997年至2005年先后任美国Arizona州立大学访问教授、加拿大纽芬兰纪念大学助理教授、副教授,从2005年9月至今任纽芬兰纪念大学教授。2008年9月获该校University Research Professorship荣誉。
赵晓强教授是国际应用动力系统和生物数学领域中最活跃的学者之一。他关于单调动力学、一致持久性、行波解和渐进传播速度、基本再生数的理论及应用方面的一系列工作已经成为了相关研究领域的经典文献。迄今为止,赵晓强教授在“Comm. Pure Appl. Math.、 J. Eur. Math. Soc.、 J. Reine Angew. Math.、 J. Math. Pures Appl.、 SIAM J. Math. Anal.、 SIAM J. Appl. Math.、J. Nonlinear Science、J. Functional Anal.、 J. Differential Equations、J. Math. Biology、Bull. Math. Biology”等国际著名期刊上发表学术论文100余篇,并著有 “Dynamical Systems in Population Biology(Springer-Verlag)”。此外,赵教授目前担任加拿大数学会主办杂志“Canadian Mathematical Bulletin”的主编和另外两个国际动力系统期刊的编委。
讲座题目: 两维及高维系统的Hopf分支理论和奇异摄动 (II)
讲座人:李承治教授, 北京大学
讲座摘要:
二维和高维的Hopf分支,主要是当某个参数在某一临界值发生变化时奇点的稳定性的变化,周期解个数的变化等。 用阿贝尔积分或奇异摄动理论研究闭轨的分支问题(快慢系统理论、环性等)。 讲解分支(从奇点出或闭轨线)产生的要点和思想,并告诉学生可在什么参考书或文章中找到细节。
讲座题目:A Linear SEIR Epidemic Model for Contact Networks
讲座人: 黄森忠教授, 南开大学 & Univ. Rostock
讲座摘要:
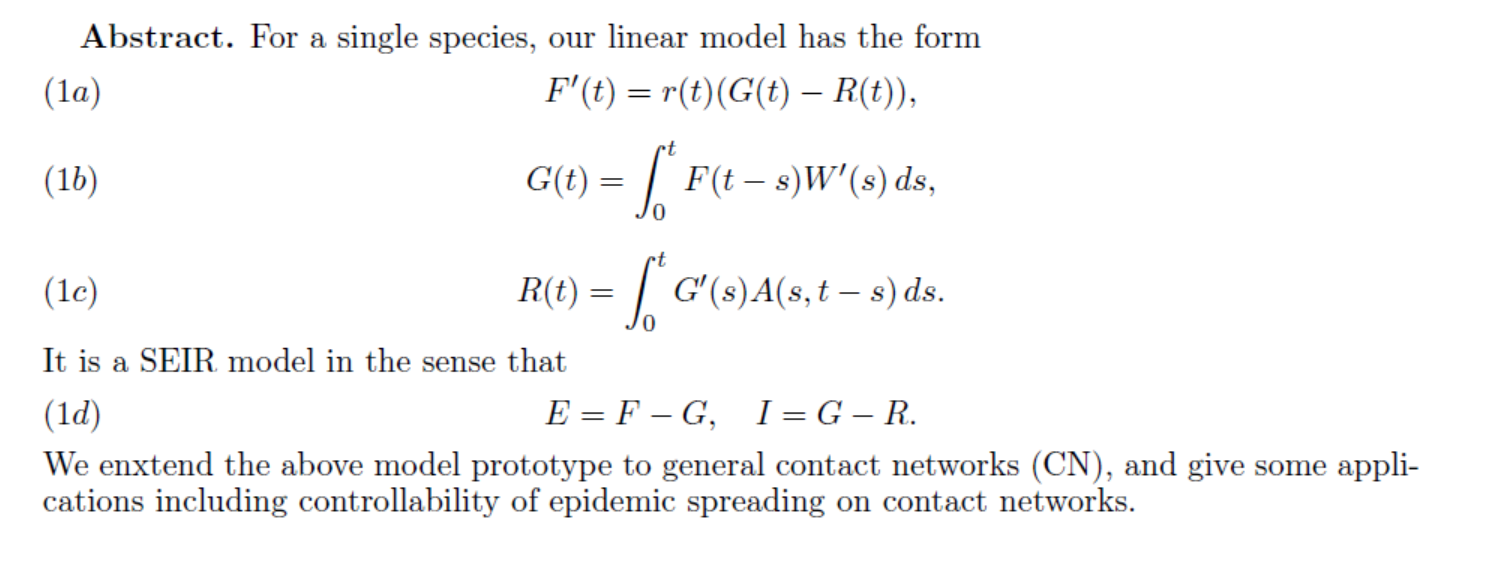
讲座人简介:
黄森忠教授分别于1983年7月和1986年7月在南开大学数学系毕业,获理学学士和理学硕士。后在南开大学任教多年。1991年赴德留学,并于1996年获得德国图宾根大学博士学位。随后(1997-1998)在德国耶拿大学做博士后进修。自1998年起在德国罗思托克大学从事研究和教学。2018年3月被聘为南开大学统计研究院客座教授及“智英健康数据研究中心”主任。主要研究方向包括应用分析特别是“数学流行病学”,非线性泛函分析及非线性发展方程。出版专著Gradient Inequalities: with applications to asymptotic behavior and stability of gradient-like systems. Mathematics Survey and Monographs Vol.126, Amer. Math. Soc. (2006)。