项目编号:地区发展活动DQ201803
会议时间:9月29日-30日
会议地点:兰州大学逸夫科学馆
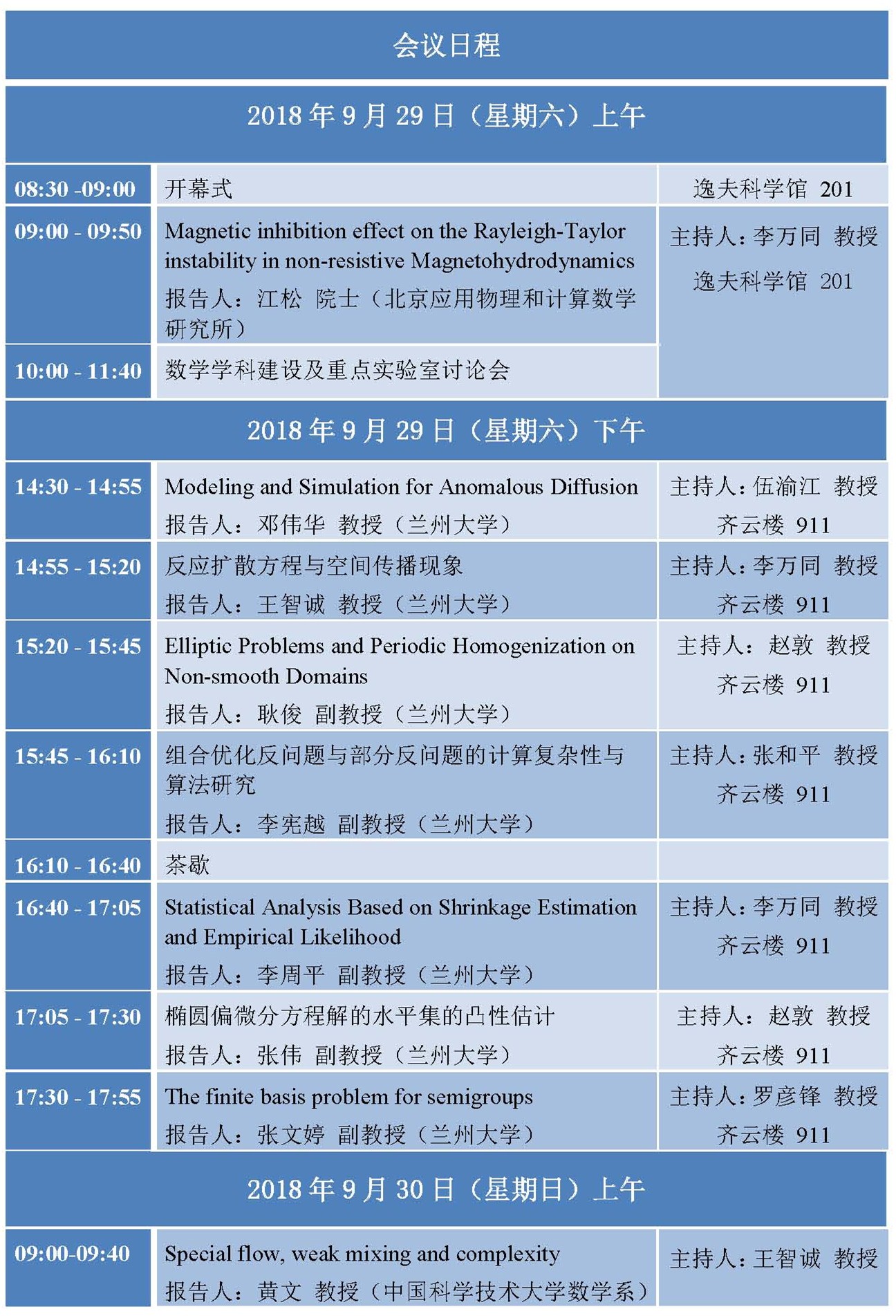
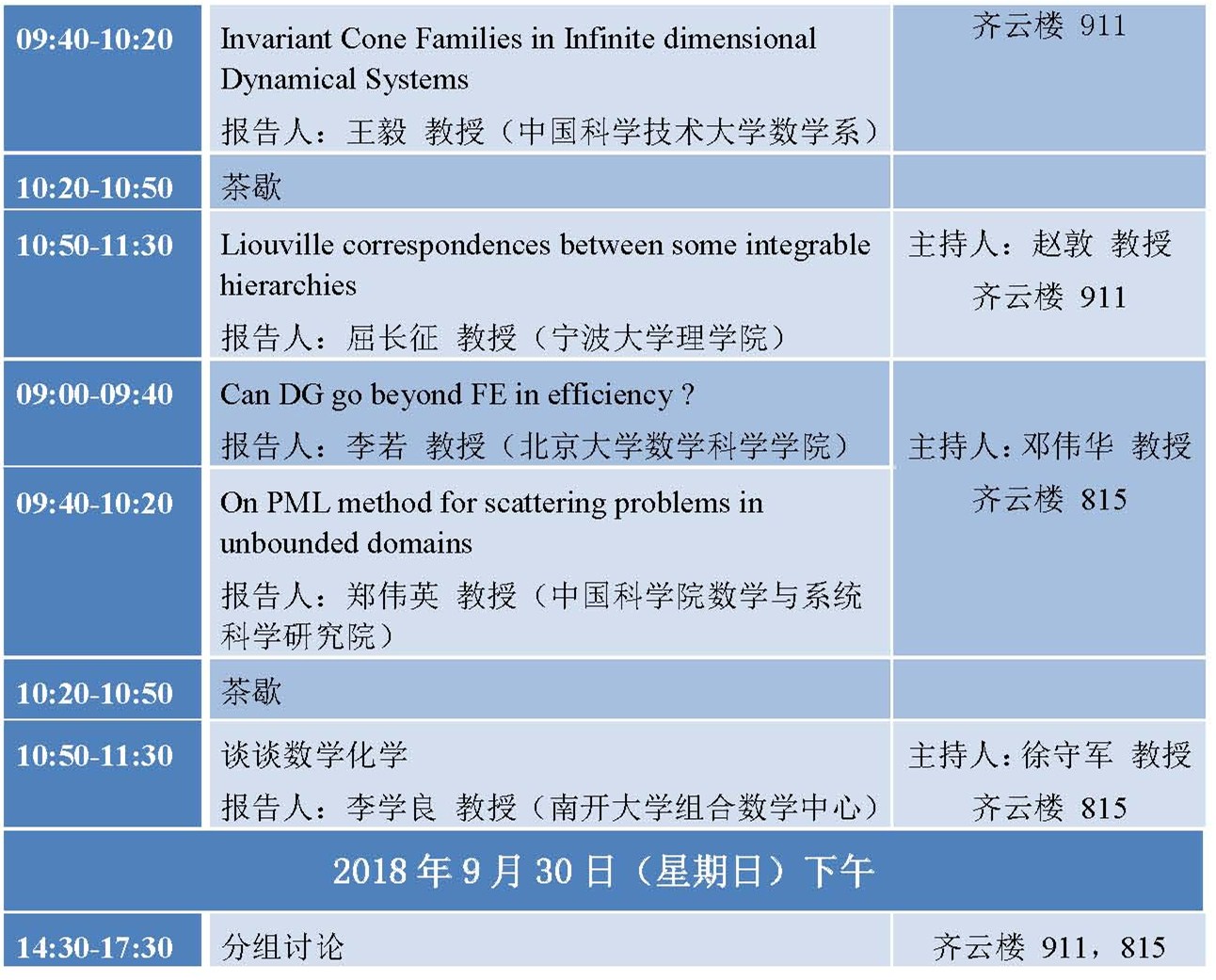
会议日程:
特邀报告
Magnetic inhibition effect on the Rayleigh-Taylor instability in non-resistive Magnetohydrodynamics
江松 院士(北京应用物理和计算数学研究所)
The Rayleigh-Taylor (RT) instability is well known as gravity-driven instability in fluids when a heavy fluid is on top of a light one. It appears in a wide range of applications in science and technology, such as in inertia confinement fusion, Tokamak, supernova explosions. In this talk, mathematical analysis of the magnetic RT instability in both incompressible and compressible fluids will be presented, in particular, effects of (impressed) magnetic fields upon the growth of the RT instability will be discussed and analyzed quantitatively. We shall show that a sufficiently strong (impressed) magnetic field can inhibit the RT instability; otherwise, instability will still occur in the sense that solutions do not continuously depend on initial data. Moreover, we shall give an explanation of physical mechanism for the magnetic inhibition phenomenon based on mathematical analysis.
Special flow, weak mixing and complexity
黄文 教授(中国科学技术大学数学系)
In this talk, we will review some progress on Sarnak’s conjecture. Finally, we will focus on the complexity of a special flow built over an irrational rotation of the unit circle and under a roof function on the unit circle. We construct a weak mixing minimal special flow with bounded topological complexity. We also prove that if the roof function is C∞, then the special flow has sub-polynomial topological complexity and the time one map meets the condition of Sarnak’s conjecture. This based on joint works with Li, Thouvenot, Wang, Xu,Ye.
Invariant Cone Families in Infinite dimensional Dynamical Systems
王毅 教授(中国科学技术大学数学系)
In this talk, we will report some recent progress on the invariant cone families (ICF) in infinite-dimensional dynamical systems. For linear cocycles, we will discuss the close relation of the ICF with Multiplicative Ergodic Theorem, dominated splitting (exponential separation), as well as Krein-Rutman Type Theorem. For nonlinear cocycles, we show that ICF plays a key role in investigating the dynamics of non-autonomous parabolic equations on the cycle. In particular, we show the appearance of almost periodically (automorphically) forced circle flow generated by these nonlinear parabolic equations.
Liouville correspondences between some integrable hierarchies
屈长征 教授(宁波大学理学院)
In this talk, we study the Liouville correspondences between the the modified CH and modified KdV hierarchies, between Novikov and Sawada-Kotera hierarchies, and between the Degasperis-Procesi and Kaup-Kupershmidt hierarchies. We show that that a pair of Liouville transformations between the isospectral problems of the Novikov and Sawada-Kotera equations, the isospectral problems of the Degasperis- Procesi and Kaup-Kupershmidt equations, and the isospectral problems of the modified CH and modified KdV equations, relate the corresponding hierarchies, in both positive and negative directions, as well as their associated conservation laws. Combining the generalized Miura-transformation relating the Sawada-Kotera and Kaup-Kupershmidt equations, we further establish implicit relationships between Novikov and Degasperis-Procesi equations, between the CH equation and modified CH equation.
Can DG go beyond FE in efficiency ?
李若 教授(北京大学数学科学学院)
The discontinuous Galerkin method has attracted tremendous amount of attentions in the last decades since it has been applied to problems with regular solutions, the 2nd order elliptic equation for example. In spite of its well-known advantages, the efficiency of discontinuous Galerkin method for problems with very regular solutions is a weak point which has often been attacked at. In this talk, I will show that the discontinuous Galerkin method may go beyond the continuous finite element method in efficiency for elliptic problems, where is the traditional area for the finite element method to outperform. Our technique to help DG out is to construct a brand-new approximate space which will be clarified in my talk.
On PML method for scattering problems in unbounded domains
郑伟英 教授(中国科学院数学与系统科学研究院)
In this lecture, I will talk about the perfectly matched layer (PML) method for acoustic and electromagnetic scattering problems. The PML method is widely used in the engineering literature and proves to be very efficient for solving wave propagating problems. Since the pioneering work of Berenger, remarkable progresses have been made in the study of PML methods for acoustic, electromagnetic, and elastic scattering problems in homogeneous background media. Two major issues to be tackled are the stability and the exponential convergence of PML solutions. However, the results for PML methods are still rare in two important application areas: (1) scattering problems in inhomogeneous media; (2) wave propagations in time domain. In this talk, I mainly focus on the stability and exponential convergence of PML methods for scattering problems in two-layer media. Moreover, some progresses and comments will also be made for time-domain PML methods.
谈谈数学化学
李学良 教授(南开大学组合数学中心)
数学化学是将数学应用到化学的一个研究领域。欧洲科学院院士Gutman和德国著名理论化学家Polansky在《Mathematical Concepts in Organic Chemistry》一书的引言中给出了一个简短而全面的定义:数学化学就是理解化学概念背后的数学结构,建立和研究化学现象的数学模型,并将数学的思想和技巧应用到化学中去。我们将对数学化学这一交叉学科做一简介。1975年诺贝尔化学奖得主Vladimir Prelog说过:“图的形象表示是如此地明白易懂使得化学家们经常仅仅满意于考察和讨论它们,却没有足够地注意到它们的代数方面,然而很明显,熟悉图的理论对于更深刻地理解它们的性质是必需的”。所以,我们将侧重于化学图论的介绍;然后,对数学化学中的主要论著以及代表性人物给出介绍;最后介绍“国际数学化学科学院(International Academy of Mathema- tical Chemistry,简称IAMC)”这一国际学术组织。